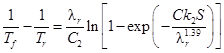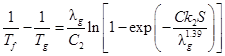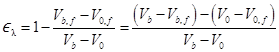THERMALRADIATION.NET

EXPERIMENTAL EXAMINATION OF SOOT CONCENTRATION

One technique to obtain information on the soot-concentration quantity Ck₂, as in Eq. (D-16) for the visible region, is to sight through the flame onto a cold black background with a pyrometer and match the brightness of the pyrometer filament to that of the flame while using a red filter and then a green filter. With each filter the pyrometer is also sighted on a blackbody source, and the source temperatures are obtained that produce the same brightness as when the flame was viewed. As a convenient simplification at the small λT for red and green wavelengths when considering typical flame temperatures, the blackbody intensity can be approximated very well by Wien’s formula, Eq. (1-20). Then, if T_r is the blackbody temperature producing the same brightness as the flame did when the red filter was used, this intensity is

                                                                 (D-16)

where λ_r = 0.665 μm. This intensity can also be written as a spectral emittance of the flame times the blackbody intensity at the flame temperature,

This yields

                                           (D-17)

Similarly, with a green filter (λ_g = 0.555 μm),

                                          (D-18)

Now, as a simple approximation, using (D-6) for k_λ in the visible region, (D-2) becomes

                                             (D-19)

where S is the path length sighted through the flame. Substitute (D-19) into (D-17) and (D-18) to obtain

                               (D-20)

                                         (D-21)

These two equations are solved for T_f and Ck₂ to yield the needed measure of the soot concentration as well as the flame temperature.

As an approximation, Ck₂ is assumed independent of wavelength and is used in (D-2), (D-4), and (D-6) to yield, for a path length S,

                                (D-22)

                                (D-23)

These spectral emittances can be used with the definition in Eq. (D-12) to evaluate the total emittance of the flame as . The hope is that the Ck₂ can be applied to “similar” flames. This is a very rough approximation; so many variables affect the flow and mixing in the flame that it is difficult to know when the flames will have a similar character. The detailed nature of flames and their radiating characteristics is a continuing area of active research [Taylor and Foster (1974), Magnussen and Hjertager (1977), Gibson and Monahan (1971), Selcuk and Siddall (1976), Kunitomo (1974), Chin and Lefebvre (1990)].

Another way to measure flame emittance is to use a radiometer and a blackbody source with a blackened chopper in front of it that periodically covers and uncovers the blackbody aperture (Fig. D-8). With the flame turned off, the voltage signal at the detector from the uncovered blackbody is V_b, and with the blackbody covered by the chopper it is V₀. With the flame turned on these signals become V_b,f and V_0,f. The measurements are made through a filter so that they are for a small spectral region Δλ centered about λ. The transmittance of a path through the flame is . Then V_b,f is the result of transmitted radiation from the blackbody and emission by the flame so . Similarly, . The two signals are subtracted, and the spectral emittance obtained for the path viewed through the flame is

                                   (D-24)

FIGURE D-8 Radiometric measurement of flame emittance.

Scattering of laser light can be used to measure local soot concentrations, as discussed in Santoro et al. (1983, 1987). Although scattering by soot is small, it can be accurately detected with sensitive instrumentation. Mie scattering theory provides the scattering intensity in a given direction as a function of soot concentration and optical properties. If values are assumed for the optical properties, the measured scattering intensities can be used to obtain local soot concentrations. Usually spherical soot particles are assumed, and the agglomeration of some of the soot is a source of error. Agglomeration was found in Ku and Shim (1991) to have little effect on the absorption coefficient, but it did influence scattering. Another difficulty is in selecting the soot complex refractive index. In Santoro at al. (1983)  was chosen for soot in an ethene flame at the argon ion laser wavelength of 0.5145 μm. The phase function for soot aggregates is in Köylü and Faeth (1996) for observations in the visible region at λ = 0.514 μm. For measuring flame temperature without soot, a two-color emission pyrometry method is in Bhattcharjee et al. (2000).