THERMALRADIATION.NET

A.5 Infinite Plate to Any Area on Parallel Plate

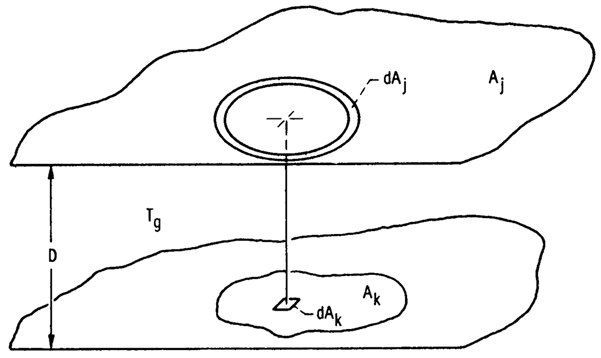
In Fig. A-5 consider on one plate an element dA_k, and on the other plate a concentric ring element dA_j centered about the normal to dA_k. The geometry is like that in Fig. A-2 for a ring on the top of a cylinder to the center of its base. Then, from (A-6),

where k_λD is the optical spacing between the plates. By using the procedure leading to (A-8), the integral is transformed to . Then integrating over any finite area A_k as in Fig. A-5 gives . With  and F_k–j = 1, this reduces to

                                                         (A-13)

FIGURE A-5 Isothermal layer of medium between infinite parallel plates.